Question
A stone is thrown from ground level over horizontal ground. It just clears three walls, the successive distances between them being r and 2r. The inner wall is 15/7 times as high as the outer walls which are equal in height. The total horizontal range is nr, where n is an integer. Find n.
Solution
Let us just assume that both the outer walls are equal in height say h and they are at equal distance x from the end points of the parabolic trajectory as can be shown below in the figure.
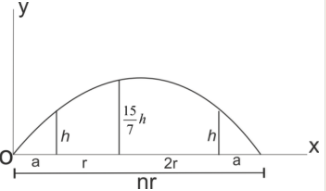
Now equation of the parabola is
y=bx−cx2 (1)
y=0 t x=nr=R
where R is the range of the parabola.
Putting these values in equation (1) we get
b=cnr (2)
Now the range RR of the parabola is
R=a+r+2r+a=nr
This gives
a=(n−3)r/2 (3)
The trajectory of the stone passes through the top of the three walls whose coordinates are
(a,h),(a+r,15h/7),(a+3r,h)
Using these co-ordinates in equation 1 we get
h=ab−ca2 (4)
15h/7=b(a+r)−c(a+r)2 (5)
h=b(a+3r)−c(a+3r)2 (6)
After combining (2), (3), (4), (5) and (6) and solving them we get n = 4.
Question
Which two quantities are constant throughout projectile motion when air resistance is negligible?
(A) The speed and acceleration.
(B) The vertical component of velocity and acceleration.
(C) The magnitude of the acceleration and the speed.
(D) The horizontal component of velocity and acceleration.
(E) The direction of the velocity and acceleration.
Solution:
(A) The speed and acceleration.
- speed is not constant. a = constant = g.
(B) The vertical component of velocity and acceleration.
- ay ≠ 0 ⇒ vy not constant.
(C) The magnitude of the acceleration and the speed.
- speed is not constant.
(D) The horizontal component of velocity and acceleration.
- ax = 0 ⇒ vx = constant.
(E) The direction of the velocity and acceleration.
- Direction of v changes.
Therefore answer is D.
Question
A truck goes around a circular track of radius R at speed v so that it makes one circuit every T seconds As it does so it experiences a centripetal acceleration of magnitude p
If the trcuk now goes around a different circular track of radius 4R so that it now takes a time ½T to go around once, what is the magnitude of its centripetal acceleration?
(A) ½ p
(B) 16p.
(C) 4p.
(D) 8p.
Solution
Initial situation
a1 = v2 /R
Now v=2πR/T
or a1= 4π2 R/T2
On the second track:
a2= 4π2 (4R)/ (.5T)2
=64π2 R/T2
Now
a2 = 16 a1 =16c
Question
A particle moves such that
x = (18.0)t
and y = 4t – 4.90t2
(a) Write a vector expression for the particle position as a function of time, using the unit vectors i and j
(b) Obtain the expression for the velocity vector as a function of time
(c) Obtain the expression for the acceleration vector a as a function of time.
(d) Find the the position, the velocity, and the acceleration of the particle at t = 1.00 s.
Solution
(a) Position vector is given by
r= xi + yj
Therefore
r= (18.0)t i + [4t – 4.90t2 ] j
(b) v= dr/dt =d[(18.0)t i + [4t – 4.90t2 ] j ]/dt =18 i + (4-9.8t)j
(c) a= dv/dt =-9.8 j
(d) At t=1 sec
r= (18.0)t i + [4t – 4.90t2 ] j
r= (18.0)i – .9 j
(e) At t = 100 s,
v=18 i + (4-9.8t)j =18i -5.8 j
(f)At 1= 3.00 s,
a = -9.8 j
A stone is thrown from ground level over horizontal ground. It just clears three walls, the successive distances between them being r and 2r. The inner wall is 15/7 times as high as the outer walls which are equal in height. The total horizontal range is nr, where n is an integer. Find n.
Solution
Let us just assume that both the outer walls are equal in height say h and they are at equal distance x from the end points of the parabolic trajectory as can be shown below in the figure.
Now equation of the parabola is
y=bx−cx2 (1)
y=0 t x=nr=R
where R is the range of the parabola.
Putting these values in equation (1) we get
b=cnr (2)
Now the range RR of the parabola is
R=a+r+2r+a=nr
This gives
a=(n−3)r/2 (3)
The trajectory of the stone passes through the top of the three walls whose coordinates are
(a,h),(a+r,15h/7),(a+3r,h)
Using these co-ordinates in equation 1 we get
h=ab−ca2 (4)
15h/7=b(a+r)−c(a+r)2 (5)
h=b(a+3r)−c(a+3r)2 (6)
After combining (2), (3), (4), (5) and (6) and solving them we get n = 4.
Question
Which two quantities are constant throughout projectile motion when air resistance is negligible?
(A) The speed and acceleration.
(B) The vertical component of velocity and acceleration.
(C) The magnitude of the acceleration and the speed.
(D) The horizontal component of velocity and acceleration.
(E) The direction of the velocity and acceleration.
Solution:
(A) The speed and acceleration.
- speed is not constant. a = constant = g.
(B) The vertical component of velocity and acceleration.
- ay ≠ 0 ⇒ vy not constant.
(C) The magnitude of the acceleration and the speed.
- speed is not constant.
(D) The horizontal component of velocity and acceleration.
- ax = 0 ⇒ vx = constant.
(E) The direction of the velocity and acceleration.
- Direction of v changes.
Therefore answer is D.
Question
A truck goes around a circular track of radius R at speed v so that it makes one circuit every T seconds As it does so it experiences a centripetal acceleration of magnitude p
If the trcuk now goes around a different circular track of radius 4R so that it now takes a time ½T to go around once, what is the magnitude of its centripetal acceleration?
(A) ½ p
(B) 16p.
(C) 4p.
(D) 8p.
Solution
Initial situation
a1 = v2 /R
Now v=2πR/T
or a1= 4π2 R/T2
On the second track:
a2= 4π2 (4R)/ (.5T)2
=64π2 R/T2
Now
a2 = 16 a1 =16c
Question
A particle moves such that
x = (18.0)t
and y = 4t – 4.90t2
(a) Write a vector expression for the particle position as a function of time, using the unit vectors i and j
(b) Obtain the expression for the velocity vector as a function of time
(c) Obtain the expression for the acceleration vector a as a function of time.
(d) Find the the position, the velocity, and the acceleration of the particle at t = 1.00 s.
Solution
(a) Position vector is given by
r= xi + yj
Therefore
r= (18.0)t i + [4t – 4.90t2 ] j
(b) v= dr/dt =d[(18.0)t i + [4t – 4.90t2 ] j ]/dt =18 i + (4-9.8t)j
(c) a= dv/dt =-9.8 j
(d) At t=1 sec
r= (18.0)t i + [4t – 4.90t2 ] j
r= (18.0)i – .9 j
(e) At t = 100 s,
v=18 i + (4-9.8t)j =18i -5.8 j
(f)At 1= 3.00 s,
a = -9.8 j
0 comments:
Post a Comment