3.Instantaneous velocity

3.Instantaneous velocity

• We already know that instantaneous velocity is the velocity of the particle at any instant of time or at any point of its path.
• If we bring point Q more and more closer to point P and then calculate average velocity over such a short displacement and time interval then 
  
   
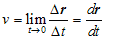
  where v is known as the instantaneous velocity of the particle.
  
• Thus, instantaneous velocity is the limiting value of average velocity as the time interval aproaches zero.
• As the point Q aproaches P, direction of vector Δr changes and aproaches to the direction of the tangent to the path at point P. So instantaneous vector at any point is tangent to the path at that point.
• Figure below shows the direction of instantaneous velocity at point P.
  
  
   
  
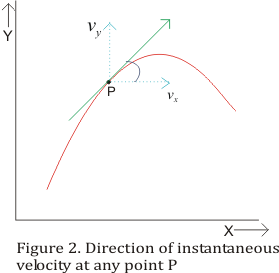
• Thus, direction of instantaneous velocity v at any point is always tangent to the path of particle at that point.
• Like average velocity we can also express instantaneous velocity in component form
  
   
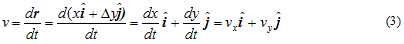
  where v_x and v_y are x and y components of instantaneous velocity.
• Magnitude of instantaneous velocity is 
  |v|=√[(v_x)²+(v_y)²]
  and angle θ which velocity vector makes with x-axis is
  tanθ=v_x/v_y
• Expression for instantaneous velocity is
  
   
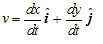
  Thus, if expression for the co-ordinates x and y are known as function of time then we can use equations derived above to find x and y components of velocity.